An elevator (lift) consists of the elevator cage and a counter weight, of mass m each. The cage and the counter weight are connected by chain that passes over a pulley. The pulley is coupled to a motor. It is desired that the elevator should have a maximum stopping time of t seconds from a peak speed V. If the inertias of the pulley and the chain are neglected, the minimum power that the motor must have is
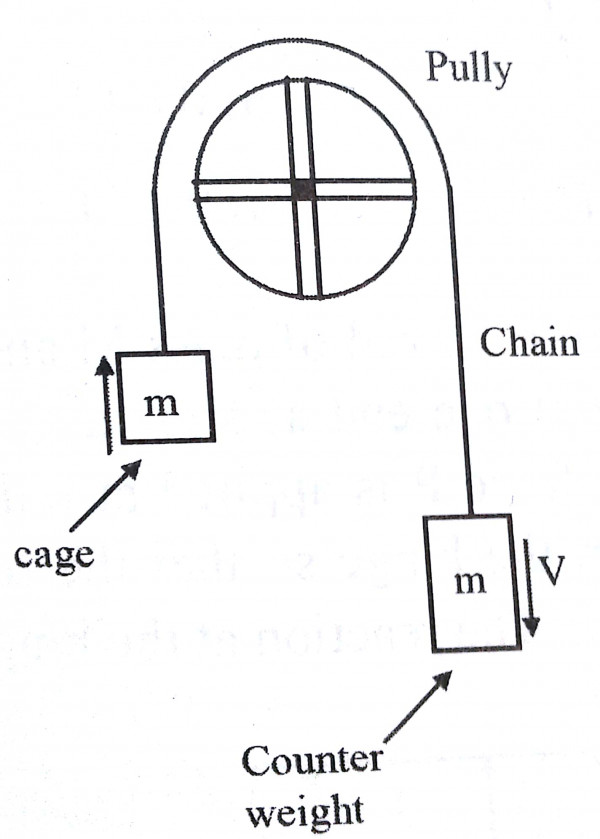
(a) 
$\frac{1}{2}mv^{2}$ (b)$\frac{mv^{2}}{2t}$ 
(c) $\frac{mv^{2}}{t}$ 
(d) $\frac{2mv^{2}}{t}$ 